

Derivative of log 2x how to#
See change of base rule to see how to work out such constants on your calculator.) derivative of 2x2-5x-3 Pre Algebra Algebra Pre Calculus Calculus Functions Matrices & Vectors Geometry Trigonometry Statistics Physics Chemistry Finance Conversions full pad.

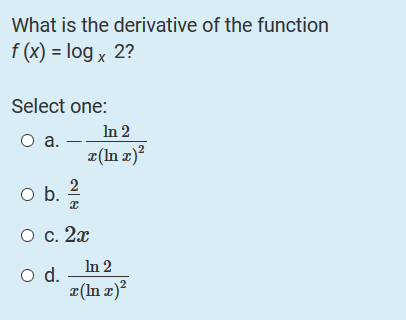
Then we can obtain the derivative of the logarithm function with base b using: `=2\ cot\ 2x+x/(x^2+1)` Differentiating Logarithmic Functions with Bases other than e In the particular case, the derivative is given by. Suppose we are given a pair of mutually inverse functions and Then. `(dy)/(dx)=(2\ cos 2x)/(sin 2x)+1/2 (2x)/(x^2+1)` As the logarithmic function with base, and exponential function with the same base form a pair of mutually inverse functions, the derivative of the logarithmic function can also be found using the inverse function theorem. Next, we use the following rule (twice) to differentiate the two log terms: asked Apr 10 in Differentiation by Takshii (35.0k points) closed Apr 12 by Takshii. It means the same thing.įirst, we use the following log laws to simplify our logarithm expression: We need the following formula to solve such problems. For example, we may need to find the derivative of y = 2 ln (3 x 2 − 1). This derivative can be found using both the definition of the derivative and a calculator. Most often, we need to find the derivative of a logarithm of some function of x. The derivative of the natural logarithmic function (lnx) is simply 1 divided by x. Unfortunately, we can only use the logarithm laws to help us in a limited number of logarithm differentiation question types. log e x is frequently denoted lnx, and called the natural logarithm. For example, log 2 8 3 since 23 8 and log 3 1 3 1 since 3 1 3. Derivative of y = ln u (where u is a function of x) Section 11.1, Derivatives of Logarithmic Functions 1 Quick Review of Logarithms For a constant a with a > 0 and a 6 1, recall that for x > 0, y log a x if ay x. The above graph only shows the positive arm for simplicity. NOTE: The graph of `y=ln(x^2)` actually has 2 "arms", one on the negative side and one on the positive. The graph of `y=ln(x^2)` (in green) and `y=ln(x)` (in gray) showing their tangents at `x=2.` The graph on the right demonstrates that as `t->0`, the graph of `y=(1+t)^` is:ġ 2 3 4 5 6 7 -1 1 2 3 -1 -2 -3 -4 x y slope = 1 slope = 1/2 Open image in a new page 1 2 3 4 5 -1 -2 2 4 6 8 10 -2 t y e Open image in a new page


 0 kommentar(er)
0 kommentar(er)
